Table of Contents
What is flow in fluid dynamics

In fluid dynamics, “flow” refers to the motion of a fluid, which can be a liquid or a gas. Fluid flow is a fundamental concept in physics and engineering that involves the study of how fluids move, interact, and behave under different conditions. Understanding fluid flow is crucial in various applications, such as designing efficient transportation systems, predicting weather patterns, and optimizing industrial processes.
Fluid dynamics utilizes mathematical equations, such as the Navier-Stokes equations, to describe the behavior of fluid flow. Solving these equations is often complex and requires numerical simulations for real-world applications.
Flow phenomena in fluid dynamics can be quite intricate, and researchers and engineers continuously study and model fluid flow to improve our understanding and develop practical solutions to various engineering and environmental challenges.
Properties of fluid flow
In fluid dynamics, the properties of fluid flow refer to the characteristics and parameters that describe how a fluid moves and behaves. Understanding these properties is essential for analyzing and predicting fluid behavior in various applications. Some of the key properties of fluid flow include:
Velocity
Velocity is the rate of change of position of a fluid particle with respect to time. It is a vector quantity, meaning it has both magnitude and direction. In fluid dynamics, velocity provides information about how fast and in which direction the fluid is moving at any given point.
Pressure
Pressure is the force exerted by the fluid per unit area. It is a scalar quantity and is essential for understanding the distribution of forces within the fluid. Pressure variations in fluid flow can affect the flow patterns and play a crucial role in the behavior of the fluid.
Density
Density is the mass of a fluid per unit volume. It is a fundamental property of the fluid and determines its response to external forces, such as gravity. In fluid dynamics, density is used in equations to describe the conservation of mass and other fluid flow phenomena.
Temperature
Temperature is a measure of the average kinetic energy of the fluid particles. It can significantly influence fluid flow behavior, especially in cases where thermal effects are important, such as in natural convection or heat transfer processes.
Viscosity
Viscosity is a measure of a fluid’s resistance to flow or internal friction. Fluids with high viscosity (e.g., honey) flow more slowly and with more resistance, while fluids with low viscosity (e.g., water) flow more easily. Viscosity plays a critical role in determining whether flow is laminar or turbulent.
Compressibility
Compressibility is a measure of how much a fluid’s volume changes under pressure variations. Incompressible fluids have negligible volume changes, while compressible fluids experience significant volume changes with changes in pressure and temperature.
Reynolds Number (Re)
The Reynolds number is a dimensionless parameter used to predict the flow regime of a fluid (i.e., laminar or turbulent). It is determined by the fluid’s density, velocity, characteristic length, and viscosity.
Mach Number (Ma)
The Mach number is another dimensionless parameter, specifically relevant for compressible flow, and represents the ratio of the fluid’s velocity to the local speed of sound. It is used to characterize the flow regimes, such as subsonic, transonic, and supersonic.
Streamlines and Flow Patterns
Streamlines are imaginary lines drawn in a fluid flow to represent the instantaneous velocity direction at various points. Flow patterns describe how the fluid moves and interacts with obstacles or boundaries, such as separation, vortices, and eddies.
These properties, among others, are fundamental to fluid dynamics and are used in mathematical equations and models to describe and analyze fluid flow behavior in various engineering and scientific applications.
Type of flow
In the context of fluid dynamics, there are several types of flow that describe the behavior and characteristics of fluid motion. Here are some common types of flow:
Laminar Flow
In laminar flow, fluid particles move smoothly in parallel layers or streams without significant mixing or crossing over. The flow is characterized by a steady and organized pattern with minimal turbulence.
Turbulent Flow
Turbulent flow is characterized by chaotic, irregular fluid motion with fluctuations in velocity, pressure, and direction. Fluid particles mix vigorously, leading to the formation of eddies and vortices. Turbulent flow is often observed at high velocities or in the presence of obstacles and irregularities.
Transitional Flow
Transitional flow is a combination of laminar and turbulent flow characteristics. It occurs during the transition between laminar and turbulent states and is characterized by intermittent turbulence and flow instability.
Steady Flow
In steady flow, the velocity, pressure, and other flow properties remain constant at any given point over time. The flow pattern does not change with time.
Unsteady Flow
Unsteady flow, also known as transient flow, refers to fluid motion where the velocity, pressure, and other flow properties vary with time. The flow pattern changes over time, and there may be fluctuations and disturbances in the flow.
Compressible Flow
Compressible flow occurs when the density of the fluid changes significantly due to changes in pressure and temperature. This type of flow is relevant in the study of gases and high-speed fluid dynamics.
Incompressible Flow
In incompressible flow, the density of the fluid remains constant, and changes in pressure and temperature have a negligible effect on the flow properties. Liquids are often considered incompressible in most practical scenarios.
Subsonic and Supersonic Flow
Subsonic flow refers to fluid motion where the velocity of the fluid is lower than the speed of sound in that medium. Supersonic flow occurs when the fluid velocity exceeds the speed of sound. The behavior and characteristics of flow are significantly different in these two regimes.
These are some of the common types of flow encountered in fluid dynamics. The choice of the flow type depends on the specific conditions and applications being studied.
Laminar Flow
Laminar flow refers to a type of fluid motion where the fluid particles move smoothly in parallel layers or streams without significant mixing or crossing over. In this flow regime, the fluid flows in an organized and predictable manner, with each layer of fluid maintaining its own velocity profile. Laminar flow is characterized by the following features:
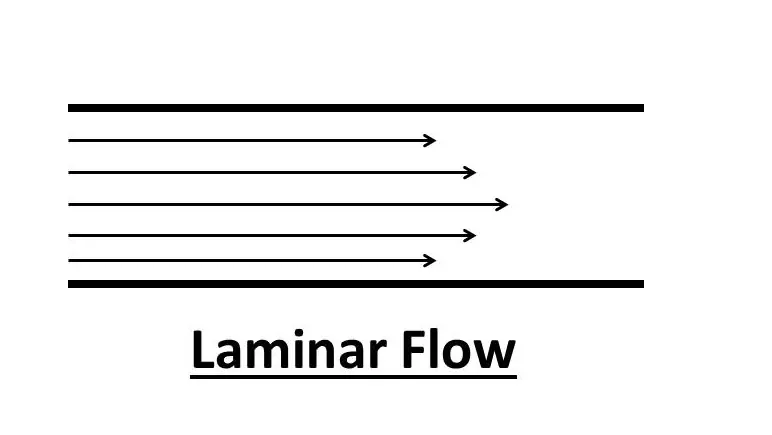
Smooth Streamlines: The fluid particles move in well-defined paths called streamlines, which do not intersect or mix with each other. Each streamline represents a layer of fluid moving with a consistent velocity.
Low Turbulence: Laminar flow exhibits minimal turbulence, with fluid particles moving in a highly ordered manner. The velocity gradients within the flow are relatively small, resulting in low levels of mixing and disturbances.
Predictable Flow Patterns: Laminar flow follows a predictable pattern, often described as “plug flow” or “parabolic flow” in pipe-like geometries. The velocity profile across the flow is typically uniform, with the maximum velocity at the center and gradually decreasing towards the edges.
Low Energy Dissipation: Laminar flow experiences minimal energy losses due to fluid friction. The frictional forces between adjacent layers of fluid are relatively small, resulting in lower pressure drops along the flow direction.
Reversible Flow: Laminar flow is reversible, meaning that if the flow direction is reversed, the flow pattern will remain unchanged. The streamlines would simply mirror their original path.
Laminar flow is often observed at low velocities, in small-scale systems, or in situations where the fluid has a low viscosity. It can be seen in various natural and engineered systems, such as slow-moving rivers, blood flow in capillaries, and certain industrial processes. Understanding laminar flow is crucial for designing efficient fluid transport systems, optimizing heat transfer, and studying fluid behavior in various scientific and engineering applications.
Turbulent Flow
Turbulent flow refers to a type of fluid motion characterized by chaotic, irregular fluctuations in velocity, pressure, and direction. Unlike laminar flow, turbulent flow is highly disordered, with fluid particles mixing vigorously and forming eddies and vortices. Turbulent flow exhibits the following features:
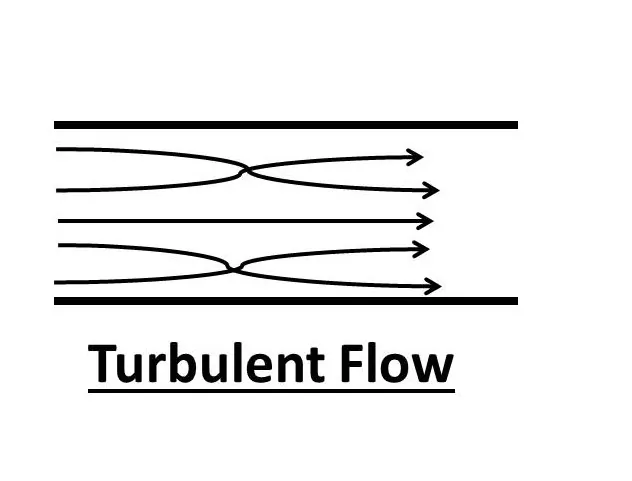
Random and Chaotic Motion: In turbulent flow, fluid particles move in an erratic and unpredictable manner. The velocity and direction of individual particles vary significantly, leading to a lack of well-defined streamlines.
Mixing and Diffusion: Turbulent flow promotes the mixing of fluid particles, resulting in a thorough blending of different regions of the fluid. This mixing enhances the transfer of momentum, heat, and mass between different parts of the flow, leading to efficient mixing and diffusion processes.
Turbulent Structures: Turbulent flow is characterized by the presence of coherent structures, such as eddies and vortices, which span different scales. These structures contribute to the transfer of energy and momentum within the flow.
High Energy Dissipation: Turbulent flow experiences significant energy losses due to fluid friction. The irregular motion of fluid particles generates turbulent eddies, which result in increased frictional forces and higher pressure drops along the flow direction.
Enhanced Heat and Mass Transfer: Turbulent flow promotes efficient heat and mass transfer due to enhanced mixing. This makes it particularly useful in applications such as industrial mixing, combustion processes, and convective heat transfer.
Sensitivity to Initial Conditions: Turbulent flow is highly sensitive to initial conditions and small perturbations. Even tiny changes in flow conditions or geometry can lead to significant alterations in the turbulent behavior.
Turbulent flow occurs at higher velocities or in the presence of obstacles and irregularities that disrupt the smooth laminar flow. It is commonly observed in natural phenomena such as rivers, waterfalls, ocean currents, and atmospheric flows.
Transitional Flow
Transitional flow refers to a type of fluid motion that occurs during the transition between laminar flow and turbulent flow regimes. It is characterized by intermittent turbulence and flow instability, with characteristics of both laminar and turbulent flows. Transitional flow exhibits the following features:

Fluctuating Flow Properties: In transitional flow, the velocity, pressure, and other flow properties exhibit fluctuations and variations. The flow can switch between laminar-like and turbulent-like behavior intermittently.
Flow Instabilities: Transitional flow is associated with flow instabilities, which can cause the formation and propagation of small-scale vortices or disturbances within the flow. These instabilities can lead to localized turbulence and mixing.
Unpredictable Behavior: Transitional flow is less predictable compared to laminar flow. The flow patterns and characteristics can vary spatially and temporally, making it challenging to determine the exact behavior of the flow.
Sensitivity to Perturbations: Transitional flow is sensitive to external influences and perturbations. Even small changes in flow conditions, geometry, or disturbances can trigger the transition from laminar to turbulent flow or vice versa.
Gradual Transition: Transitional flow represents an intermediate state between laminar and turbulent flows. As the flow conditions change, the flow may gradually transition from laminar to turbulent or vice versa, exhibiting characteristics of both flow regimes.
Turbulent Spot: A common feature of transitional flow is the formation of a localized region of turbulence within an otherwise laminar flow. This region, known as a “turbulent spot,” grows and propagates downstream, causing the transition to fully turbulent flow.
Transitional flow can occur in various fluid systems and scenarios, such as flows in pipes, boundary layers, jets, and wakes.
Steady Flow
Steady flow refers to a type of fluid motion where the velocity, pressure, and other flow properties remain constant at any given point over time. In steady flow, the flow pattern and flow properties do not change with time, even though the fluid particles continue to move.

Key features of steady flow include
Constant Flow Properties: In steady flow, the velocity, pressure, temperature, and other flow properties are uniform and remain unchanged at any specific location in the fluid domain. This means that the flow properties do not vary with time.
Time-Invariant Streamlines: Steady flow is characterized by well-defined streamlines that remain unchanged over time. Streamlines represent the paths followed by fluid particles, and in steady flow, these paths are fixed and do not shift or deform.
Conservation of Mass and Energy: Steady flow implies that the mass and energy entering a particular region of the flow are balanced by the mass and energy leaving that region. This conservation principle allows for the analysis of steady flow using mathematical equations such as the continuity equation and energy equation.
Predictable Flow Behavior: Steady flow exhibits a predictable and consistent behavior, making it easier to analyze and model. The flow properties can be determined at any point in the flow domain without the need to consider changes over time.
Steady flow is often assumed in many engineering applications, such as the design of pipelines, channels, and steady-state heat transfer systems. It simplifies the analysis and calculations, as the time dependence of flow properties is neglected. However, it is important to note that in reality, most flows exhibit some degree of unsteadiness, even if it is negligible.
It’s worth mentioning that steady flow does not imply that the fluid is stationary or not moving. The fluid particles can still be in motion, but the flow properties at any specific point do not change with time in steady flow.
Unsteady Flow
Unsteady flow, also known as transient flow, refers to a type of fluid motion where the velocity, pressure, and other flow properties vary with time. In unsteady flow, the flow pattern and flow properties change over time, and the behavior of the fluid particles is not constant.

Characteristics of unsteady flow include
Time-Varying Flow Properties: In unsteady flow, the velocity, pressure, temperature, and other flow properties are not constant at a given location. These properties change with time, reflecting the dynamic nature of the flow.
Changing Streamlines: Unlike steady flow, unsteady flow is associated with changing streamlines. The paths followed by fluid particles, represented by streamlines, are not fixed and may shift or deform as the flow evolves.
Transient Phenomena: Unsteady flow often involves transient phenomena, such as flow initiation, flow shutdown, or flow disturbances propagating through the system. These transient effects introduce temporal variations in the flow properties.
Unpredictable Flow Behavior: Unsteady flow is more complex and unpredictable compared to steady flow. The flow properties at a specific location cannot be determined without considering the changes over time. The behavior of the flow may depend on initial conditions, boundary conditions, and disturbances.
Time-Dependent Conservation Laws: In unsteady flow, the conservation equations for mass, momentum, and energy involve time derivatives, accounting for the temporal changes in flow properties. These equations need to be solved in conjunction with appropriate initial and boundary conditions to analyze unsteady flow.
Unsteady flow is common in many real-world scenarios, such as fluid transients in pipelines, flow fluctuations in natural streams, unsteady combustion processes, and unsteady aerodynamic phenomena. Understanding and analyzing unsteady flow is crucial for predicting system response, assessing dynamic effects, and designing systems that can handle transient conditions.
It is important to note that the distinction between steady and unsteady flow is not always clear-cut, as some flows may exhibit a combination of steady and unsteady characteristics. In such cases, a transient analysis may be required to capture the complete flow behavior.
Compressible Flow
Compressible flow refers to the behavior of a fluid in which the density changes significantly due to changes in pressure and temperature. Unlike incompressible flow, where the density remains relatively constant, compressible flow involves variations in fluid density and, consequently, other flow properties as well.

Key features of compressible flow include
Density Variations: In compressible flow, changes in pressure and temperature cause the fluid density to change. This density variation affects other flow properties, such as velocity, temperature, and pressure.
Changes in Flow Area: Compressible flow often involves changes in the flow area, such as through nozzles, diffusers, or other flow restrictions. The area changes result in changes in flow velocity, density, and pressure, following the principles of mass conservation and continuity equation.
Significant Effects of Mach Number: The Mach number, defined as the ratio of the flow velocity to the speed of sound, plays a crucial role in compressible flow. When the flow velocity approaches or exceeds the speed of sound, compressibility effects become more pronounced, leading to phenomena such as shock waves and sonic flow.
Energy Exchange: Compressible flow involves energy exchange between kinetic energy, potential energy, and internal energy due to changes in fluid density and velocity. This energy exchange can lead to changes in flow properties and affect the overall system performance.
Wave Propagation: Compressible flow supports the propagation of pressure waves, which can be observed as shock waves, expansion waves, or acoustic waves. These waves transmit changes in flow properties and play a significant role in compressible flow phenomena.
Compressible flow is prevalent in various applications, including aerodynamics, gas dynamics, jet engines, supersonic and hypersonic flows, rocket propulsion, and high-speed fluid systems. Understanding the behavior of compressible flow is essential for designing efficient and safe systems in these fields.
Analyzing compressible flow requires the consideration of additional equations, such as the compressible form of the continuity equation, momentum equation, and energy equation, which account for density variations and the associated changes in flow properties. Numerical methods and computational fluid dynamics (CFD) techniques are often employed to simulate and analyze compressible flow phenomena accurately.
Incompressible Flow
Incompressible flow refers to the behavior of a fluid in which the density remains nearly constant regardless of changes in pressure and temperature. In an incompressible flow, the density variation is negligible compared to other flow properties, and the fluid can be treated as effectively incompressible.

Key features of incompressible flow include
Constant Density: In incompressible flow, the density of the fluid is considered constant or nearly constant. The density variations due to changes in pressure or temperature are typically insignificant and can be ignored for most practical purposes.
Conservation of Mass: In an incompressible flow, mass is conserved according to the continuity equation. The mass flow rate remains constant throughout the flow field, and the fluid cannot be created or destroyed within the system.
Simplified Equations: Incompressible flow allows for the simplification of governing equations. For instance, the continuity equation reduces to a simpler form, and terms related to density variation can be neglected in the momentum and energy equations.
Streamline Conservation: In incompressible flow, streamlines represent the paths followed by fluid particles. Streamlines are conserved and do not intersect or mix with each other. This allows for simplified visualization and analysis of the flow behavior.
Low Mach Number: In incompressible flow, the flow velocity is much lower than the speed of sound in the fluid medium. Consequently, compressibility effects, such as shock waves and sonic flow, can be disregarded.
Incompressible flow is commonly encountered in many fluid systems, including most liquid flows, flows involving low velocities, and flows with small pressure variations. Examples of incompressible flows include water flow in pipes, air flow at low speeds, and blood flow in human circulatory systems.
Analyzing incompressible flow involves solving simplified equations, such as the incompressible form of the continuity equation (based on mass conservation) and the Navier-Stokes equations (based on momentum conservation). These simplified equations can be solved analytically or numerically using various techniques, such as finite difference, finite element, or computational fluid dynamics (CFD) methods.
Subsonic and Supersonic Flow
Subsonic and supersonic flow are two distinct regimes of fluid motion characterized by different relative velocities to the speed of sound in the medium. The speed of sound is the speed at which pressure disturbances propagate through a fluid.
Subsonic Flow
Subsonic flow refers to fluid motion where the velocity of the fluid is lower than the speed of sound in that medium. In subsonic flow, the fluid particles move at speeds below the speed of sound, resulting in smooth and predictable flow patterns. The Mach number, defined as the ratio of the flow velocity to the speed of sound, is less than 1 in subsonic flow (M < 1).
In subsonic flow, the fluid behaves in accordance with the laws of incompressible flow or, more precisely, the incompressible flow assumptions can be applied with a reasonable degree of accuracy. The flow properties, such as velocity, pressure, and density, change relatively slowly, and the flow can be analyzed using simplified equations based on the conservation of mass and momentum. Common examples of subsonic flows include air flowing around most everyday objects or water flowing through pipes at typical speeds.
Supersonic Flow
Supersonic flow, on the other hand, refers to fluid motion where the velocity of the fluid exceeds the speed of sound in the medium. In supersonic flow, fluid particles move faster than the speed of sound, leading to a different set of flow characteristics. The Mach number in supersonic flow is greater than 1 (M > 1).
Supersonic flow is associated with compressible flow effects and exhibits several unique phenomena, such as shock waves and expansion waves. Shock waves form when supersonic flow encounters obstacles or undergoes significant changes in flow properties, causing abrupt changes in pressure, temperature, and density. Expansion waves occur when supersonic flow decelerates and transitions to subsonic speeds.
Analyzing supersonic flow requires the consideration of compressible flow equations, such as the full compressible Navier-Stokes equations or the Euler equations. These equations account for the density variations and changes in flow properties associated with supersonic flow. Examples of supersonic flows include the exhaust gases from rocket nozzles, high-speed aircraft, and the bow shock wave formed by a moving object in a fluid.
FAQ
What are the factors that affect fluid flow?
Factors that affect fluid flow are fluid viscosity, fluid density, and fluid velocity.
Write the formula for the mass flow rate.
The formula for the mass flow is given by:
Mass flow = ρAV
You amy also like
